ENERGY FIELDS EFFECTS IN INTERNAL COMBUSTION ENGINES

Dr. Stephen C. Bates

Thoughtventions Unlimited LLC

40 Nutmeg Lane

Glastonbury, CT 06033

1. Introduction

The object of this research has been: 1) To provide a detailed prescription for achieving a significant performance improvement for a specific internal combustion engine through the application of an energy field inside the cylinder, and 2) To Describe a detailed scientific explanation of the physical effects that cause this improvement. It is the intent of the overall ORNL project to experimentally and theoretically demonstrate this improvement.

It cannot be overemphasized that any internal combustion engine experiment that simply puts a widget on the engine and tries to see what effect it has on engine operation will find the effect to be bad. The reason for this is that the operating conditions of the engine have been carefully set up for the specific control, combustion, and geometry of that engine that creates optimum combustion. This does not imply that the engine cannot be improved, but that engine experiments that test improvements must be carefully designed at specific conditions to determine whether the "improvement" results in a real beneficial effect. Such an effect usually applies to one operating regime where engine performance is normally poor.

Once a novel engine control technique has been demonstrated in a research environment the technology must be transferred to the automotive industry. A simple presentation of results will not initiate an industry program to put the improvement in a car. The constraints of the economics and manufacturability of auto components are severe, the sophistication of the current technology is not great, and the awareness of and belief in the possibilities of technological innovation are very limited. Perhaps the most difficult barrier to overcome is the belief by industrial (and research) automotive engineers that all the radical concepts for combustion control have been tried and have failed. The failures of these concepts result from 1) There is no overall improvement in engine performance, 2) The concept is not practical energetically (too large an energy input is required), or 3) It is impractical or uneconomical to install the device in a production vehicle. Any contacts in the auto industry must be made with these facts in mind.

Microwaves in Engines - Microwave enhancement of combustion has been repeatedly attempted in a research environment with consistent results that do not justify commercial development. All of the above drawbacks have been true for combustion enhancement using microwaves up to this time. There is also a generic belief that the use of microwaves is not cost effective unless there is no competing technology (as in microwave ovens).

2. Laminar Hydrocarbon Flames

There are some useful generalizations that can be made about laminar hydrocarbon flames. First, molecular species diffusion depends on the species molecular weight such that heavier species diffuse more slowly. This means that low mass species such as H, H₂, O, and OH will diffuse most rapidly. In particular, the high diffusion rate of H atoms is responsible for many important phenomena connected with laminar flames. Second, transport coefficients generally increase with increasing temperature and decrease with increases in density. These overall trends have a considerable effect on the variation of laminar burning velocity with pressure and unburned gas temperature.

The diffusion of hydrogen atoms into the preheat zone is the primary triggering mechanism for the chain- branching reactions which support flame propagation. The hydrogen atom undergoes two primary competing reactions during any hydrocarbon oxidation process. These are the chain-branching reaction

H + O₂ → OH + O           (1)

and the recombination reaction

H + O₂ + M → HO₂ + M           (2)

Furthermore, the chain-branching reaction has a first-order pressure sensitivity (because it is a second-order reaction) and has a large activation energy, which means that its rate increases very rapidly with increased temperature. Conversely, the recombination reaction has a second-order pressure sensitivity (because it is a third-order reaction) and has essentially no temperature sensitivity at all. In the preheat zone of the flame, hydrogen atoms diffuse toward the cold gas and at the same time react. In the higher temperature regions of the flame, the chain-branching reaction dominates and recombination is slow. However, as the H atoms move toward the incoming gas the temperature drops and at some point the two reactions become competitive. In the colder regions the recombination reaction becomes dominant and destroys hydrogen atoms.

For any flame system and where the flame temperature is lowered by dilution with an inert, the burning velocity will be lower and the point in the flame at which the rate of the recombination reaction and chain-branching reaction are equal will move toward the hot boundary. For a specific mixture which has a low burning velocity and a low flame temperature if the pressure level at which the flame is burning is increased, the rate of the recombination reactions relative to the chain-branching reactions will increase and tend to lower the burning velocity. Thus for low-burning-velocity flames in which the competition between recombination and chain-branching reactions in the preheat zone is important, there will be a negative pressure exponent.

Increasing the initial temperature of the mixture should cause a marked increase in burning velocity for low- temperature flames and, because of the recombination/chain-branching competition in the preheat zone, this effect should be very strong for flames that have very low burning velocities. It should be moderately strong for flames that have burning velocities in the range of about 0.4 to 1.0 m/s and should have almost no influence on flames that have burning velocities above about 1 m/s. This is because the high effective heat capacities of the dissociated product gases at these high temperatures will cause the flame temperature and, therefore, the burning velocity, to change only very slightly as the initial temperature of the mixture is changed.

A guide to the variation in flame parameters with pressure and flame velocity is given in Table 1. It should be noted that there is a strong dependence on the initial temperature of the gas, which is important for internal engine combustion, since the air/fuel mixture is heated by compression.

Table 1. The dependence of flame parameters on pressure and flame velocity [1].

Variable	Dependence
Burning velocity	P-1/4
Flame thickness	P-1, v0-1
Residence time	P-1, v0-2
Maximum logarithmic gradient	P, v0
Maximum reaction rate	P2, v02
Maximum heat release rate	P2, v02

The variation of flame speed with fuel composition for combustion in air is shown in Fig. 1. This graph provides an indication of the magnitudes of typical flame speeds, and shows how unusual some fuels are. Gasoline is a complex mixture of many components and has a fairly low flame speed, on the order of 20 cm/sec.

Experimental studies of real flames and theoretical modeling with relatively complete kinetic schemes [2] have shown that in addition to the structural features of a flame illustrated by the

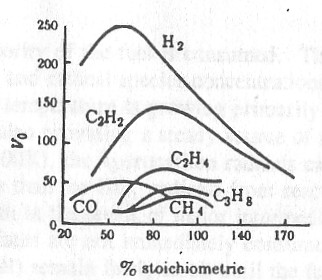
Figure 1. Flame velocities of some typical flames in air. [3]

simpler thermal and A-B reaction theories real flames contain a high-temperature region where the reactions slowly reach equilibrium Simply stated, the final oxidation of carbon monoxide to carbon dioxide occurs primarily through the exchange reaction

CO + OH → CO₂ + H           (3)

which is relatively slow. Therefore in most flame systems the carbon monoxide concentration goes through a maximum and finally decays to its equilibrium value somewhat downstream of the rapid reaction region of the flame. Since the oxidation of CO to produce CO₂ is exothermic this final equilibration causes the temperature to rise downstream of the fast reaction zone.

Three-body reactions are required for the attainment of equilibrium in any flame which involves radicals, as most flames do. Flames at atmospheric pressure usually separate into an initial narrow, fast reaction where bimolecular reactions dominate, and a wide, slow secondary reaction zone where termolecular reactions reestablish equilibrium in radical concentrations. Below 0.1 atm, termolecular reactions are insignificant. Above 1000 atm, they dominate. [1]

There are distinct spatial regions in a laminar, premixed flame in which generically different processes take place. These regions are described as follows:

Region 1:

Well ahead of the flame the temperature is low and the unreacted fuel and air are relatively inert.

Region 2:

An induction region (1.0 - 3.0 mm at 1 atm) extends in front of the flame where the gas temperature is slowly increasing as a result of thermal conduction from the hot flame nearby. Very little heating from chemical reactions occurs. A radical pool is growing by species diffusion from the flame, but because the temperature is very low chain branching does not occur. Reactions between fuel molecules and radical species, particularly H atoms begin the process of fuel consumption. Hydrogen atoms also recombine with O2 molecules to form HO2, leading to fairly large amounts of H₂O₂

H + O₂ + M = HO₂ + M           (4)

HO₂ + HO₂ = H₂O₂ + O₂           (5)

C_nH_m + HO₂ = C_nH_m-1 + H₂O₂           (6)

Recombination is favored over branching for H + O₂ in this part of the flame because E₂ = -1.0 kcal/mol, and the low temperature does not affect reaction (4).

Region 3:

In this region the majority of the fuel is consumed. The principal reactions are as in Region 2, but the higher temperature and radical species concentrations make the fuel consumption rate much faster than in Region 2. The temperature is growing primarily as a result of thermal diffusion from the hot flame zone, which is also providing a steady source of radical species. Because the temperature is higher (400-1200K), the hydrocarbon radicals can now decompose, providing H atoms which are much more reactive than the HO₂ radicals from reaction (6). The fuel consumption is accompanied by a rapid growth in the levels of major intermediate species, including fuel fragments, H₂, and CO. These intermediates are not immediately consumed because the radical species concentrations (particularly OH) remain fairly small until the fuel has all disappeared. The inhibition of CO and H₂ oxidation by the hydrocarbon molecule should be emphasized. Approximately half of the total temperature increase occurs in Region 3, resulting in large part from the production of CO.

Region 4:

Region 4 is characterized by the rapid growth in radical concentration once all of the fuel has been consumed. This region extends from -0.2 to -1.0 mm and is described by the elementary reactions of the H₂-CO-O₂ system. Most of the remaining temperature increase occurs in this region.

Region 5:

Region 5 is the burned gas product region, where a slow approach to final chemical equilibrium takes place, involving the termolecular recombination reactions. The oxidation of CO to CO₂ is another slow reaction that takes place in this region.

Figure 2. Concentration profiles in a 0.1 atm methane-oxygen flame. [4]

Figures 2 and 3 show the variation of the various species in a typical flame. The upstream side is characterized by decreasing fuel and oxygen, the flame center contains the maximum concentration of radical species which denote the peak reaction zone, and the post flame gases are characterized by the slow conversion of carbon monoxide.

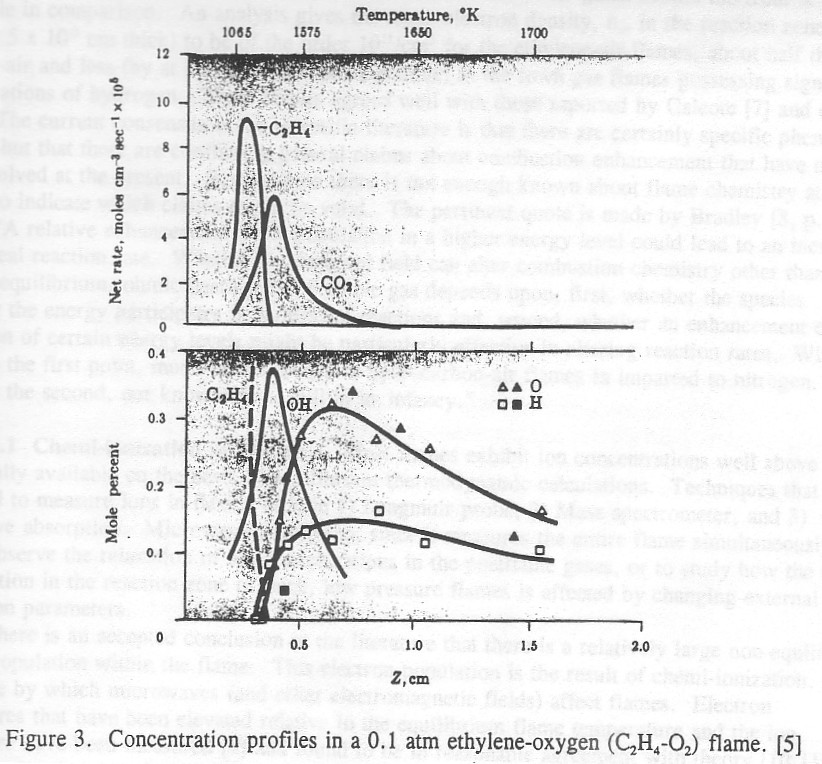
Figure 3. Concentration profiles in a 0.1 atm ethylene-oxygen (C₂H₄-O₂) flame. [5]

3. The Effects of Microwaves on Laminar Flames

It is important to identify the route of the microwave energy dissipation in the combustion gas, as well as the effects of this energy transfer process. The goals of this effort are to:

• Determine the primary energy cascade between the absorption of the microwaves and their final thermalization.
• Determine if there are specific mechanisms that would cause the deposition of microwave energy to result in enhancement of combustion chemistry.
• Predict the effects of these mechanisms and determine how they can be used to advantage to control combustion or pollution in an internal combustion engine.
• Determine if there are specific power regimes where microwave excitation is either effective or ineffective for engine combustion enhancement.

It is established that the primary absorption of microwave energy in flames is in the flame front itself, where the electron density is anomalously high as a result of chemi-ionization. Measurements of the current through a flame propagating through a tube obtained for different dc voltages and mixtures by Jaggers and von Engel [6] showed that the entire current passes through the narrow flame front, since the conductivity of the extensive hot flame gases behind the front is negligible in comparison. An analysis gives the mean electron density, n_e, in the reaction zone (taken as being 5 x 10^-2 cm thick) to be of the order 10¹¹/cm²³ for the ethylene-air flames, about half this for methane-air and less (by at least an order of magnitude) in the town gas flames possessing significant concentrations of hydrogen. These results agreed well with those reported by Calcote [7] and others.

The current consensus in the scientific literature is that there are certainly specific phenomena present, but that there are conflicting general claims about combustion enhancement that have not been resolved at the present. Furthermore there is not enough known about flame chemistry at present to indicate which claims might be valid. The pertinent quote is made by Bradley [8, p. 374]:

"A relative enhancement of the population in a higher energy level could lead to an increase in chemical reaction rate. Whether an electrical field can alter combustion chemistry other than by general, equilibrium, ohmic heating of the whole gas depends upon, first, whether the species receiving the energy participates in significant reactions and, second, whether an enhancement of the population of certain energy levels might be particularly effective in altering reaction rates. With regard to the first point, much of the energy in hydrocarbon-air flames in imparted to nitrogen. With regard to the second, our knowledge is still in its infancy."

3.1 Chemi-ionization in Flames - Most flames exhibit ion concentrations well above those theoretically available on the basis of equilibrium thermodynamic calculations. Techniques that have been used to measure ions in flames include 1) Langmuir probe, 2) Mass spectrometer, and 3) Microwave absorption. Microwave absorption, since it measures the entire flame simultaneously, is used to observe the relaxation of ion concentrations in the postflame gases, or to study how the ion concentration in the reaction zone of thick, low pressure flames is affected by changing external combustion parameters.

There is an accepted conclusion in the literature that there is a relatively large non-equilibrium electron population within the flame. This electron population is the result of chemi-ionization, and is the avenue by which microwaves (and other electromagnetic fields) affect flames. Electron temperatures that have been elevated relative to the equilibrium flame temperature and the ion temperature have been measured [9] and found to be in reasonable agreement with theory [10,11]. This data was taken in a fuel rich propane-air flame (φ = 1.2) for a nominal 500 W of power at 2.5 GHz and 50 % duty cycle (60 Hz). Flame electron temperatures were measured as 0.34 eV, or about 4000 K with a Langmuir probe. Post-flame gases had an electron temperature measured even higher, at 0.5 eV, or 5800 K [9]. It is not at all clear what causes the post-flame heating, because the electron density is much lower than in the flame. It is possible that the equilibrium electron density is sufficient to provide a means for the slight additional heating, while energy losses are small.

It is thought [12] that the primary ion-producing reaction in hydrocarbon flames is the reaction:

CH + O → CHO⁺ + e^-           (7)

The production of other ions are thought to be formed by a series of charge exchange reactions with either this ion or with free electrons. This mechanism of primary ion formation also explains the observation that hydrogen-oxygen flames have extremely low ion concentrations relative to the hydrocarbon flames of much lower temperature. Even though ionization occurs in flames, flame structure calculations and measurements of neutral species concentrations agree reasonably well without the inclusions of ionization processes. This implies that ionization reactions are dead- end reactions in the chemical sense.

Fristrom and Westenberg [1] state that a flame is a weak plasma with a peak electron mole fraction approximately 10^-7, which, for a gas at 2000 K and 1 atm., would imply an electron density of approximately 4 x 10¹¹. Electron densities for various flames have been quoted in the literature. Jaggers and von Engel [6] quote an electron density of 10^-11 /cm³ for stoichiometric ethylene-air flames. Wortberg [13] measured a peak value in a equivalence ratio, φ, of 0.51 methane-air flame of about 1.8 x 10^-10 /cm³. McIntosch and Clarke [14] measured peak value in a φ = 1.0 propane-air flame of 3.5 x 10^-11 /cm³. These are all consistent measurements and lead to a confident knowledge of flame electron densities for a variety of flames.

Plasma Location - If reaction (7) is the primary source of electrons in the flame, conclusions can be drawn about the specific location of the most of the electron density in a laminar flame.

Assuming reaction (7) to be the source of the electrons, then the region of maximum electron density will be where there is a spatial maximum in the product of the O and CH radical concentrations. Since this reaction is dependent on collision frequency there may also be a displacement from the concentration product maximum by an increase in the collision frequency caused by a significantly higher temperature nearby that might more than compensate for the decreased radical population.

In terms of the laminar flame regions discussed previously, one can immediately exclude Regions 1 and 2 from having significant electron density because of the low temperature and minimal radical populations. In Region 3 the temperature and radical concentrations are growing, but intermediate species are not consumed because radical concentrations remain fairly small (particularly OH) until the fuel is consumed [2]. CO and H₂ oxidation are inhibited by the preference for available hydrocarbon molecules. Approximately half of the total temperature increase occurs within Region 3, mostly as a result of the production of carbon monoxide. In this region the low concentration of OH also implies a low concentration of O, since of the dominant reactions that produce O (listed by Westbrook and Dryer [2]), that with the lowest activation energy (by far) is

H + HO₂ → H₂O + O (E_a = 1.0)           (8)

Other reactions that proceed simultaneous with this reaction are

H + HO₂ → OH + OH (E_a = 1.9)           (8)

and

H + HO₂ → H₂ + O₂ (E_a = 0.7)           (10)

such that O radicals always have a lower concentration than OH radicals.

Reaction (8) implies that electron production simultaneously requires the existence of CH radicals, which are oxidized in the flame as one of the final intermediate species of the degradation of the fuel. The reactions given by Westbrook and Dryer [2] that produce CH are:

CH₂ + O → CH + OH (E_a = 25.0)           (11)

CH₂ + OH → CH + H₂O (E_a = 25.70)           (12)

CH₂ + H → CH + H₂ (E_a = 25.70)           (13)

where the dominant reaction is:

CH₂ + O → CO + CH (E_a = 0.0)           (14)

Consumption reactions for CH are given as:

CH + O₂ → HCO + O (E_a = 0.0)           (15)

CH + O₂ → CO + OH (E_a = 25.7)           (16)

From the above reactions and their activation energies, it can be concluded that O atoms both produce and consume CH radicals, and at the same time produce the electron plasma. Thus, at the edge of Region 4 near the flame center, the concentration of O atoms is decreasing from a maximum behind the flame, and the concentration of CH radicals is decreasing from a maximum nearby towards the front of the flame. The electron density is at its maximum where the product maximizes, near the center of the flame. Charge neutrality requirements ensure that the region of increased electron concentration is coincident with it production.

This argument implies that the electron plasma is concentrated in a narrow region around the flame center. At this location the fuel is nearly completely consumed, and elementary reactions of the CH, C₂, H₂, CO, and O₂ system are taking place, at a location very close to the maximum heat release in the flame.

Plasma Layer Thickness - The flame thickness for a laminar, stoichiometric propane flame at one atmosphere pressure is approximately 1 cm [1]. This thickness, t, decreases with pressure (t ∝ 1/p), but increases for leaner mixtures (t ∝ 1/v_f, v_f = flame speed). Fristrom and Westenberg [1] cite ion species measurements (p. 226) from which the approximate thickness of the ionized region can be calculated as approximately 1/3 the nominal flame thickness. Translating this to engine practice, the flame is turbulent but is generally considered to be a convoluted laminar flame. For a 9 to 1 compression ratio, and considering for 30 degrees before TDC (soon after lean ignition), the pressure will be approximately 7 atm without significant combustion compression, and the electron plasma thickness will be on the order of 0.5 mm. It is thus to be understood that microwave energy transfer is only a volume energy transfer process in the sense that turbulence distributes the flame front, and that this front travels rapidly through the volume as a result of fluid dynamic processes.

Note for Sooting Flames - It is also believed that the nucleation process of soot involves ion reactions and that the ion species are also formed primarily by the reaction

CH + O → CHO⁺ + e^-

followed by reactions of the type

CHO⁺ + C₂H₂ → C₂H₃⁺ + CO           (17)

which then lead to rapid ionic polymerization. These ions grow in size until they become incipient particles with atomic masses of about 10⁴. They then form crystallites and finally spherical particles whose masses range from 10⁵ to 10⁷. These soot spheres have diameters in the 10 to 50 nm range. After they are formed they aggregate to form chains. In a premixed sooting flame this all happens in about 10 to 50 ms. [12]

3.2 Microwave Absorption and Energy Transfer in Flames - The Microwave Energy Cascade; Summary - Research since the proceeding progress report has indicated that the physical processes involved in the energy transfer from the microwave electromagnetic fields to the combustion gases can be defined and quantified based on published work. The energy cascade process begins when the microwaves create high frequency large amplitude electric fields in the gas from which electrons (but not ions) can absorb energy. The electrons are created by chemi-ionization in a narrow region of the flame front where the flame radical species concentrations that contribute to the ionization reaction are large. The ratio of neutrals to radicals to ions is approximately 1:10^-2:10^-7 in an atmospheric pressure flame. Ionization in the flame has also been shown to be irrelevant to important flame processes. The electrons are heated by the microwave radiation to a separate equilibrium temperature significantly higher than the temperature of the surrounding local flame gases. These electrons transfer their excess energy to surrounding neutral molecules by collision.

The collisional energy exchange takes place almost entirely to the vibrational states of certain neutral species as a result of 1) the large difference in mass of the colliding species, 2) a close match between a vibrational energy level of the recipient species and the average electron energy, and 3) other factors that lead to quantitative electron-neutral collision cross-sections. As a result of a large collision cross section and a dominant concentration in air-fuel combustion, electron excitation of nitrogen is by far the largest sink for the microwave supplied energy. The excited lowest vibrational state of nitrogen cannot de-excite itself because the molecule lacks an electric multipole moment, so it is de-excited through collisions with other species that can quickly convert vibrational energy to translational energy. In the case of combustion the dominant quenching species is water, which is typically at a 10% concentration at the center of the flame. The energy cascade process that has just been outlined determines the ability of microwaves to provide excited species that might enhance combustion chemistry, or the ability of the microwaves to provide ohmic heating, depending on the details of the calculations and perhaps on the parameters of the application of the microwaves.

Absorbed Microwave Power Pressure Dependence - An important question with respect to microwave absorption in engines in comparison with atmospheric laminar flames is the possible increase in absorbed power that can be achieved as a result of the higher pressure. The electron density, n_e, increases in proportion to pressure, as does the breakdown voltage, so it is expected that the maximum possible deposited power per unit volume should increase with the square of the pressure. A pressure increase also causes a proportional decrease in the flame thickness, δ_f.

δ_f ∝ 1/p           (18)

n_e ∝ p           (19)

Assuming the absorbed microwave power is proportional to electron density, and again that the maximum voltage and power, P_max, that can be applied is limited by gas breakdown, then

P_max ∝ p           (20)

P_{max abs} ∝ (P_max)(n_e) ∝ p²           (21)

For burnt gas heating, the energy deposited per unit mass determines the final gas temperature, and this varies in proportion to pressure, assuming, once again, that breakdown is the limiting factor on voltage in achieving significant combustion modification.

Considering direct effects on the flame itself, absorbed microwave power can result in both ohmic heating (leading to an expansion effect similar to burn gas heating) and reaction rate enhancement. Ohmic heating will result in an increased flame velocity in proportion to the relative increase in final gas temperature. This effect should be proportional to pressure, just as in the case of burnt gas heating, since the energy density is again the important quantity.

In the case of enhancement of the chemical reaction rate, as discussed in Groff and Krage [15] the laminar flame velocity is expected to be proportional to the square root of the reaction rate. This reaction rate is expected to greatly increase in the presence of excited molecular oxygen, leading to an increase in flame velocity. Since this is a non-linear effect, there is strong reason to believe that the increase in reaction rate will be dependent on the absolute concentration of excited oxygen, where this concentration depends on p². The flame speed will depend less strongly on pressure as a result of the square root dependence on reaction rate, but the dependence will be at least linear with pressure, as opposed to the case of normal laminar flames where the flame speed changes in proportion to p^1/4.

The details of microwave absorption theory are given by Bradley and Ibrahim [10,11], who give the power density, W_e, of absorbed microwaves as

W_e = (0.76e²/m_e)(E_rms/n_e)²(n_e)(f(ε))           (22)

Where Erms is the root mean square value of the applied field, e is the electron charge, me is the mass of the electron, and ε is the electron energy. Note the dependence of W_e on (E_rms)², a powerful motivation for maximizing the applied voltage. For a breakdown potential this is proportional to pressure and since n_e is also proportional to pressure, then

W_{e, max} ∝ p           (23)

confirming the above discussion for the case of burnt gas or flame ohmic heating.

Electron Temperature Physics - Although the correct analysis of the electron temperature and energy can only be done numerically including all of the relevant molecular energy levels and collision cross sections as performed by Bradley and Ibrahim [10,11], the effects that contribute the electron heating can be understood through a simpler model.

The electron temperature, T_e, is raised above T_g by absorption of energy from the applied microwave fields. Rate of loss of energy by collisions with the gas molecules

Collisional energy loss = 3/2[E_c(v_me/L_e)k(T_e - T_g)]           (24)

when:

v_d = drift velocity of electrons
v_me = mean random velocity of electrons
L_e = electron mean free path
E_c = average fractional energy loss per collision

Equating the power input with the power lost through collisions:

eEv_d = 3/2[E_c(v_me/L_e)k(T_e - T_g)]           (25)

T_e - T_g = 2eEv_dL_e/(3E_cv_mek)           (26)

This model gives an electron temperature on the order of an eV, as is seen in practice, but the actual T_e is actually controlled by the relevant vibrational energy level.

3.3. Molecular Excitation Effects on Combustion Chemistry - The electron collision processes in a molecular gas are, in decreasing order of electron threshold energies: 1) Ionization, 2) Dissociation, 3) Electronic excitation, 4) Vibrational excitation, and 5) Rotational excitation. There are also a variety of also attachment processes. The relative importance of these processes depends on the mean electron energy, collision cross sections, and lifetimes of the species involved.

Dissociation by electron collisions can be shown to be insignificant in comparison with that produced chemically in the flame; the same is true of electronic excitation. The rate of ionization may, under some conditions, become comparable with the natural rate, but this does not lead to significant effects in the flame, or account for an increase in the flame speed, S_u [16]. Rotational energy may also be discounted because normal molecular collisions convert any excess of rotational energy quickly into translational energy [17]., and the available rate of excitation by electron collisions cannot materially alter the concentrations of such states because of this. However, rotational excitation may well be the main energy loss process limiting the value of T_e. Translational (temperature) excitation is negligible as a result of the large difference in masses of the colliding species.

The electron-collision cross sections for the formation of higher vibrational states (energy 0.1-10 eV) are , in general, large, of the order 10^-16 cm², since these are often excited by intermediaries, namely, short-lived negative ions. Deactivation by molecular collisions is far less efficient than that of rotational states, so that an appreciable excess population may be formed [17,18].

The rate of vibrational excitation from the v = 0 to the v = 1 state of a molecule is

d/dt(M₁) = n_eM₀<q₀₁v_e>           (27)

where M₁ and M₀ are the concentrations of molecules in the v = 1 and v = 0 states, respectively, and q₀₁ is the excitation cross section by electrons. Thus the change in the number concentration of molecules excited to v = 1 from v = 0 as the gas passes through the reaction zone is

Δ M₁ = integral 0-tau (n_eM₀<q₀₁v_e>)dt           (28)

Where tau is the residence time of the molecules in the reaction zone. When the integral is replaced by mean values averaged over the electron velocity distribution throughout the zone, Eq. 4 becomes

Δ M₁/M₀ ≈ mean(n_eq₀₁v_e) x tau           (29)

This gives the change in the v = 1 population, neglecting deactivation processes. In shock tubes at atmospheric pressure and gas temperatures up to 5000 K it has been found that the vibrational temperature may be close to T_e for many molecular gases while the translational and rotational degrees of freedom remain in equilibrium [19,20]. In the reaction zone of flames the same may be the case; there is not sufficient time for equilibrium to be established. Few data are presently available on the vibrational excitation cross sections of flame species such as hydroxyl and hydrocarbon radicals. These are most likely to behave in a similar way.

Vibrationally excited molecules in general can lose this energy either by collision or by radiation. However, simple gases such as are only present in a flame (except CO₂) cannot radiate from vibrationally excited states because these states have no electric dipole moments. This does not apply to the electronically excited states of CH, C₂, and OH radicals that dominate flame radiation. Another factor in the energy transfer process is that resonant transfer processes that might result in increased cross-sections are not important at flame temperatures.

The work of Bradley and Ibrahim [10,11], among others, has shown that nitrogen is the primary sink of electron energy unless very high average energies can be obtained before collisional excitation of a neutral occurs. This is because the excitation cross sections for nitrogen are significantly larger than the other flame species (Fig. 4), and because the gas is 80% nitrogen. The first vibrational level of nitrogen is at 0.3 eV, such that this energy should act as a ceiling to the average electron energy in the flame. The existence of such an electron energy ceiling is confirmed by the sole electron temperature measurement of a microwave heated flame by MacLatchy [9], which gave a T_e value of 0.34 eV. The dominance of nitrogen as an electron energy sink implies that vibrational excitation of molecules that participate in the important chemical reactions in the flame will only occur if 1) the active molecules are collisionally excited by excited nitrogen molecules (as in a CO₂ laser), 2) the electron energy input is high enough to excite species other than nitrogen in spite of the electron power loss to nitrogen excitation, or 3) the nitrogen electron energy loss sink is saturated such that the electron average energy can increase linearly with time to a level that will excite other species. The last two cases are not identical; the last case assumes that the nitrogen de- excitation is sufficiently slow so that once enough energy is deposited into the nitrogen molecule population to saturate the first vibrational level of this population, all the energy will go into increasing electron energy and exciting other species. This power level is much less than the power needed to increase the average energy in the presence of the nitrogen energy sink. It should be noted that the calculations of Bradley and Ibrahim assume that nitrogen de-excitation is fast compared with its excitation.

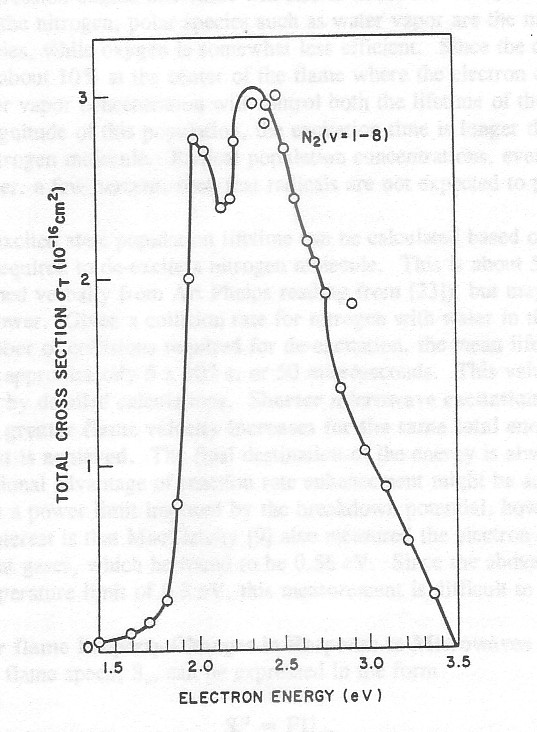
Figure 4. Total effective cross section for vibrational excitation of N₂ (ν = 1-8) by electron impact. [21]

If combustion enhancement by increasing chemical reactions is desired, the goal of microwave electron excitation should probably be to achieve significant populations of excited molecular oxygen. The reason for this is that there is experimental evidence that excited oxygen will increase the rate of formation of oxygen radicals which will in turn increase the overall hydrocarbon reaction rate in a flame because of the central nature of oxygen radicals as a kinetic limiting chain branching reaction step. The specific reaction involved is

H + O₂ = OH + O           (30)

with the understanding that the creation of OH and O radicals are the primary routes to creating H radicals. This reaction is greatly enhanced by vibrational excitation of molecular oxygen [22], as would be expected by the highly endothermic nature of the reaction. Furthermore, vibrationally excited oxygen has a very high frequency (vibration modes typically oscillate at 10¹² to 10¹⁴ Hz), and such a state will increase the reaction rate because the vibration spends most of its time at either end of the vibration, which is the highest energy state. A minor factor for this microwave process is that an increase in this reaction rate also increases ne, which depends on the concentration of CH and O radicals.

In flames at 1 atm the collision rate is about 2 x 10⁹ s^-1. At the time of early flame kernel growth in a high compression engine this value will rise to about 1 x 10¹⁰ s^-1. Various species collisionally de-excite the nitrogen; polar species such as water vapor are the most efficient, followed closely by radical species, while oxygen is somewhat less efficient. Since the concentration of both oxygen and water are about 10% at the center of the flame where the electron density is high, it is expected that this water vapor concentration will control both the lifetime of the nitrogen excited state population and the magnitude of this population, the excitation time is longer than the average de-excitation time of a nitrogen molecule. Radical population concentrations, even at the flame center, are a factor of ten lower, a few percent, such that radicals are not expected to play a significant role in deexcitation.

The nitrogen excited state population lifetime can be calculated based on the average number of collisions that are required to de-excite a nitrogen molecule. This is about 5 x 10⁴ collisions at 1500 K (a value obtained verbally from Art Phelps reading from [23]), but may be an order of magnitude higher or lower. Given a collision rate for nitrogen with water in the flame of 1 x 10⁹ s^-1, and the necessary number of collisions required for de- excitation, the mean lifetime of the excited nitrogen population is approximately 5 x 10^-5 s, or 50 microseconds. This value is tentative and needs to be confirmed by detailed calculations. Shorter microwave excitation pulse times than 50 µs may lead to much greater flame velocity increases for the same total energy deposited if chemical enhancement is achieved. The final destination of the energy is always a temperature increase, but the additional advantage of reaction rate enhancement might be achieved by using very short pulses. There is a power limit imposed by the breakdown potential, however.

One note of interest is that MacClatchy [9] also measured the electron temperature behind the flame front in the burnt gases, which he found to be 0.56 eV. Since the above discussion seems to imply an electron temperature limit of 0.3 eV, this measurement is difficult to explain.

3.4. Laminar flame Property Changes in Response to Microwaves - In all theories of flame propagation the flame speed, S_u, can be expressed in the form

S_u² = FU_ave           (31)

where F is a function of the transport coefficients and flame temperature, and U_ave is the average rate of reaction in the flame front. For a long time [24] it has been assumed that molecules react more readily if vibrationally excited. Recent theoretical work by Polanyi [25,26] shows this to be so in the case of endothermic reactions: the reaction cross section increases rapidly with increasing vibrational energy in the reactants and is relatively insensitive to increases in the translational energy. The converse is shown to hold for exothermic reactions, though the increase with translational energy is not nearly so rapid as with vibrational energy in the former case. Thus, if the vibrational energy is increased in the reaction zone of a flame, ultimately caused by a rise in T_e, then U_ave of Eq. 31 will rise while F remains essentially constant.

In methane-air flames the initial attack on the fuel is mainly by hydroxyl radicals or hydrogen atoms under fuel lean and fuel rich conditions, respectively, both fast reactions with low activation energies. The overall rate of reaction is probably controlled by the slow chain-branching reaction

H + O₂       OH + O           (32)

with high activation energy of about 16.5 kcal/mole (0.7 eV) [27].

Assume that U_ave is proportional to the average rate of Eq. 7 and that in zero field this is equivalent to a mean temperature T_o, that is,

U_ave proportional to d[OH]/dt = A[H][O₂]exp(-16.5/RT_o)           (33)

where the gas constant R = 1.99 x 10^-3 kcal/mole-K, and A is the frequency factor. In effect, T_o is considered to be the vibrational temperature of the oxygen molecules. In an electric field the vibrational energy will be increased, corresponding to a higher temperature T₁, which can be calculated by assuming a Maxwellian distribution:

M₁/M₀ = exp(-E₁/RT_o)           (34)

Here M₁ and M₀ are the concentrations of oxygen molecules in the v = 0 and v = 1 vibrational states, respectively, and E₁ is approximately equal to 0.2 eV, the energy difference between these states. Thus, if T_o = 1700 K, a 3% increase in M₁ corresponds to T₁ = 1850 K. If S_u0 and S_u1 are the burning velocities for the temperatures T₀ and T₁, respectively, then from Eqs. 32 and 34,

(S_u1/S_u0)² = exp[(1/T_o-1/T_o)16.5/R]           (35)

Hence S_u1/S_u0 is approximately equal to 1.2, that is a 3% increase in M₁ (and a corresponding increase in the higher states) produces a 20% increase in burning velocity, about equal to the maximum observed in our experiments. In addition to Eq. 7 many other reactions in the flame front are likely to be accelerated by an increase in the vibrational energy of the reactants. Thus, if an excited population can be created, a significant increase in flame speed might be expected.

3.5 Analysis of Previous Experimental Research - Previous experimental research provides divergent views of the effectiveness of using microwaves to cause flame speed increases. A valid explanation is needed for past experiments and explanations for the effects of energy fields on combustion. Given an understanding of the energy cascade in the gas that results from microwave absorption as it is discussed above, the results of the variety of flame enhancement experiments that have already been published can be understood. The basic controversy is whether or not the flame speed increases that cannot be explained by aerodynamic effects are caused by ohmic heating or reaction rate enhancement.

The most important factor to understand about microwave absorption in air/fuel combustion is that nitrogen absorbs most of the microwave energy at lower (but not low) power levels. The energy absorbed by the nitrogen is stored as vibrational energy, and this energy causes ohmic heating of the overall gas through deexcitation and thermalization by the water in the flame. The vibrational energy levels of nitrogen are such that the average electron energy is prevented from increasing significantly beyond about 0.3 eV; this effectively prevents oxygen excitation and chemical flame enhancement, except at very high absorbed power or perhaps short pulselength.

This conclusion is consistent with results reported in the literature, which (except for the speculative conclusions of Jaggers and Von Engel [28]), indicate that small increases in flame velocity associated with the application of microwaves result from ohmic heating which causes an increase in flame temperature and thus a flame speed increase. Many spuriously large results have been reported in the area of microwave excitation of flames. The common flaw is that specific experimental configurations amplify flame speed increases caused by microwave absorption as a result of aerodynamic forces induced by electromagnetic fields or because the flame geometry has changed to increase flame area and thus flame speed. In all of the reported experiments there have been no confirmed instances of chemical enhancement of flames as a result of microwave excitation of flame species.

The first goal of analyzing past research is thus to eliminate effects not caused by microwave heating of electrons. One must only consider high frequency electromagnetic excitation - more than about 50 kHz [29]. Somewhat lower frequencies include the effects of energy transfer to ions, and much lower frequencies include the effects of induced macroscopic currents and the magnetic forces on these currents in the flame. The other major confusing effect is that of the changes in flame geometry that are caused by changes in flame speed. The set of experiments left to evaluate after these exclusions is not large. The paper by Groff and Krage provides a good discussion of these problems.

In the context of microwave absorption by electrons, the work of Bradley and Ibrahim [10,11] has shown that nitrogen is the primary sink of electron energy unless very high energies can be obtained before collisional excitation occurs. This is because the excitation cross sections for nitrogen are significantly larger than the other flame species, and because the gas is 80% nitrogen. Experiments have shown little effect on flame speed for large electric field strengths (up to 100 kV/m) and at microwave frequencies where the electrons absorb the microwave power, although an increase in electron temperature has been measured. The first vibrational level of nitrogen is at 0.3 eV, such that this energy should act as a ceiling to the average electron energy in the flame. This is confirmed by the sole electron temperature measurement of a microwave heated flame by MacLatchy [9].

The rate constants for collisional excitation of oxygen and nitrogen by electrons are given by Bradley and Ibrahim [10,11], and are such that the rate constants for oxygen excitation are approximately 2 orders of magnitude lower than those for nitrogen. This implies that a significant fraction of the nitrogen must be excited in order to excite enough oxygen molecules to affect combustion chemistry. This would explain the experimental results indicating a lack of effect on combustion chemistry.

On the basis of this analysis the concluding remarks Groff and Krage can be assessed: "The microwave-enhancement concept is not practical for automotive engine applications because

1. small increases in burning velocity (same achieved by 2-3% richening of A/F mixture
2. high power required - most dissipated in the cavity walls and associated cables
3. Cavity tuning problems caused by temperature variations and deposit buildup in the cylinder
4. Turbulent flames not enhanced
5. Electron density in lean gasoline-air flames is lower"

They conclude from these (all of which appear to be true) that heating effects probably caused by ohmic heating. They then give some arguments against the existence of chemical effects caused by hot electrons. They correctly state that enhanced chemistry is a result of excited oxygen, but go on to state reasons that excited oxygen would not be expected to occur:

1. "Excited oxygen is produced near peak of electron excitation which is near the temperature peak where rate-limiting reaction should be nearly complete"
2. "Oxygen is less available in this zone as φ approaches unity, the direction of increased burning-velocity enhancement."

Both of these statements are incorrect, since the electrons are produced where the O and CH radical concentrations are a maximum and where the reaction rates are a maximum. Their experiments are very careful and convincing, however, and do seem to indication flame speed increases are caused by ohmic heating - but only because the microwave power levels are still too low.

This statement may be surprising, since their experiments were performed at electric field intensities up to 100 kV/m, but this seems to be the case. To assess the need for extremely high fields one returns to the work of Bradley and Ibrahim [10,11]. Their analysis takes as a standard value of electric field normalized by pressure (E/p)

E/p = 10 V/cm-torr

For the 1 atm flames that have been used for microwave experiments, this implies

E = 7.6 kV/cm

or

E = 760 kV/m

If one examines their electron energy exchange rate curves, it can be seen that one only begins to increase the mean electron energy past 0.3 eV at about a factor of 10 lower electron power, or a factor of 3 lower E/p, since the dependence is on the square of E/p. This implies that one only has a chance to see excited oxygen at one atmosphere at the electric field level of 1 MV/m, or 10 kV/cm. Breakdown voltage in air is about 25 kV/cm, so it appears that chemical enhancement will occur working close to breakdown.

Almost no experiments have been done near breakdown, although the work of Clements [30] is an exception. The results of this work show larger flame speed increases, but seem to be confused by the breakdown phenomena. Groff and Krage explain their results as being a result of flame geometry propagation into a closed tube that the flame speed depends strongly on overall heating.

The overall conclusion of past research into microwave enhancement of flames is that flame speed increases are a result of ohmic heating except possibly in the case of electric field intensities near breakdown at one atmosphere pressure. The above analysis indicates that lower applied fields might result in chemical enhancement at higher pressures.

4. Improving Internal Combustion Engine Performance with Energy Fields

The overall problem of discovering applications for energy fields in engine combustion can be approached in a number of ways. One approach is to identify a mode of engine operation where one might expect significant changes caused by the field, based on some sort of global reasoning. The success of this approach would be measured by experimental verification of the postulated change in engine operation. Another approach would be to identify a specific known effect of the fields on the combustion process and then try to find a mode of engine operation where this effect will be both beneficial and measurable. Either approach would be effective if it gave results, but the second approach would provide motivation for further research even if the global engine results were not definite. That assumes one could come up with some plausible reason that the engine tests were ambiguous and an experimental plan that would demonstrate positive results later.

It must be emphasized over and over again to people not familiar with internal combustion engines that there are basic facts that cannot be avoided:

1. The flows and turbulence in the cylinder will be the primary control for the overall burn rate, not anything that is done to change the flame itself.
2. To measure a specific effect on engine combustion one must carefully choose an operating regime where the effect is large, otherwise this effect will either be masked and undetectable in the natural broad variation of combustion caused by turbulent flows, or simply be a small influence on strong turbulent combustion.
3. Combustion occurs in two phases. The first phase is known as the early flame growth and accounts for most of the volume burned. This phase is driven by the expanding gases behind the flame. The second phase accounts for most of the mass burned, and occurs as the turbulent flame burns through the compressed and heated end gases.
4. A consideration to be kept in mind is that even bad effects caused by an energy field may demonstrate an important advance in the field if these effects run counter to the current understanding of the processes involved.

The basics of applying electromagnetic fields to combustion are:

1. The route to any effect of electromagnetic field on combustion is through the existence of free electrons in the flame. These electrons result from chemi-ionization and exist at densities far greater than thermal electrons.
2. There is a strong effect of fuel type in determining the electron density in the flame. The total ion production varies linearly with the carbon concentration of the fuel-air mixture for hydrocarbon flames. [31] For fuel-rich flames excess hydrogen dramatically reduces the electron density.
3. Electron temperature measurements in flame fronts are scarce.
4. It is easy to infer an incorrect increase in burning velocity without a careful experimental setup.

For microwaves:

1. There is a threshold field strength of 15-30 kV/m, below which no effects are seen.
2. The ratio of power absorbed to power applied is usually small. In the experiments discussed in the papers the absorbed microwave power is insignificant compared with the chemical power except possibly during or just after ignition.

The energetics of a typical example of microwave heating of a laminar flame is given by Groff and Krage [15]:

• Average specific heat in the flame 1250 J/(kg-K) for an equivalence ration, φ = 0.64
• Measured mass flow rate 0.81 kg/hr
• 150 W absorbed by the cavity TM₀₁₀ at 2.5 GHz
• Calculated 21 W absorbed by the flame
• heating of reaction zone - 74 K

Consensus in the Industry - The consensus in the mainstream automotive community is that except for ignition improvement there is not enough potential for achieving benefits from using energy fields in engines to justify research in the area. The pervasive view is that all of the important ideas have already been tried unsuccessfully, and most of the current ideas are put forward by eccentric individuals that provide self-justifying, selectively supporting data who are only seeking financial backing.

Consensus in the Literature on Microwave Effects - The consensus in the automotive literature is expressed by Groff and Krage [15] - that the effect of microwave heating on laminar flames is small and a result of ohmic heating. This effect is expected to be swamped by other factors for the turbulent flames at the high pressures typical of internal combustion engines.

Specifically, the microwave enhancement concept is not practical for automotive engine applications because:

1. The measured increases in burning velocity are relatively small. Similar increases can be achieved in the absence of microwaves by a 2-3% richening of the fuel-lean mixtures.
2. Although the microwave power absorbed by the flame gases is relatively low, the total power required to produce electric field intensities on the order of 100 kV/m in an engine cylinder is much greater, since most of the incident power is dissipated as resistive losses in the cavity walls and associated cables.
3. Cavity tuning problems due to temperature variations and deposit buildup in the cylinder may be significant.
4. Turbulent flames may not be enhanced significantly by microwaves.
5. Published data indicate that electron density in lean gasoline-air flames is less than that for the fuels tested here.

Combustion Improvement Concepts - The areas of combustion where possible improvements lie are given below. It should be understood that the improvements that result from a minor change can result in a major change in overall engine operation, since the overall engine set point can be changed to be closer to optimum if it need not be prevent a specific failure mode. A specific case is that of misfires. Even a single misfire can not be tolerated during normal operation as a result of the tremendous pollution generated by that single cycle. If an engine set point is optimum, but the flows in the engine cause a misfire every 100 cycles on average, this set point can not be used. If that misfire was prevented by microwaves the set point could be used and the overall engine operation improved. There is a limit on the energy used by the energy field - a few hundred mJ per cycle if it is applied every cycle. This energy must by definition be a negligible fraction of the overall combustion energy.

Most combustion improvement research is in the area of lean burn operation where greater fuel efficiency might be obtained and pollution also lowered. Such effects can be achieved through better ignition, higher initial flame speed and a consequently shorter time to bulk burn. At this time the difficulty with lean combustion is the inability of catalytic converters to operate lean - current converters are limited by the nature of their catalytic chemistry to operate with stoichiometric combustion to be effective.

Ignition - Improvements can be made in ignition reliability under adverse conditions. Methods that could be used include ignition initiated over a larger volume than for a spark plug, and perhaps feedback control of ignition to occur only in the presence of a combustible mixture (small delays introduced)

Initial flame growth - There are two ways of decreasing initial burn time: 1) Volume ignition, and 2) Increasing the initial flame speed. The former might be achieved by a volume energy field ignition, and the latter might be achieved by heating the post-flame gas or enhancing reaction rates.

Combustion completion (partial burns) - There is a great advantage in preventing partial burns that would otherwise occur. Such partial burns cannot be tolerated because of the pollution they produce. Aside from increasing the initial flame growth rate there might be a way to speed up combustion at the end of the cycle also.

Knock - Knock is a very complicated phenomenon that involves simultaneous autoignition of the unburned end gases that have been compressed by the expanding flame front. Whether knock occurs depends on the detailed time history of the end gas. Current research indicates that the knock occurs in a 2 step chemical process with different thresholds and rate constants.

It would be worthwhile to see what effect the microwaves have on knock, although it will be hard on the engine. While one might expect microwave heating to make knock worse by adding heat, the chemistry is complex enough so that some other affected chemical/thermal phenomenon may be more important and lead to an overall improvement.

Emissions - There are three primary pollutants: HC's, CO, and NO_x. Each arises from a different source. NO_x from high temperature flames, HC's from incomplete combustion with variety of causes, and CO from incomplete combustion. Any of these reactions might be affected specifically by some change in combustion resulting from the use of an energy field. The least likely seems to be the HC's, since these are generated under conditions where there are little or no flame front electrons. However, HC's resulting from incomplete combustion may be eliminated if increasing burning velocity will do it and can be achieved. It is not clear what effect introducing electron energy has on CO, and NO_x production.

Cold start - Current engines must dump in massive amounts of gas during several cycles to be able to obtain enough fuel vapor pressure to achieve ignition. This is a major source of overall auto pollution. Any technique that ignites a cold, low vapor pressure mixture would be of great value. One possibility is again a larger volume ignition, or an ignition that also vaporizes wet fuel on a nearby wall.

Microwave Cavity Response and Power Efficiency - The Q of a particular resonance is f_o/Δf, where Δf is the full width at half maximum of the power-transmission curve of the cavity plotted as a function of frequency. The energy lost is a result of resistive losses caused by currents induced in the cavity walls and in any medium within the cavity, and to the coupling of energy out of the cavity into an external load. The unloaded Q of a cavity resonance, Q₀, depends only on the losses at the cavity walls and the resonance mode. Typical values of Q₀ range from 10⁴ to 3 x 10⁴ for brass cavity walls and are about a factor of 2 larger for silver-plated cavity walls.

Quality Factor, Q:

Q = 2π x energy stored / average power dissipated           (36)

5. Effects of Other Energy Fields on In-cylinder Internal Combustion Engine Operation

VUV/UV Radiation - There are at least two possible benefits of short wavelength radiant energy ignition: larger volume ignition and low energy ignition.

The first is larger volume ignition (compared to a spark plug). This would provide a means for igniting a mixture even when there were local spots within the volume that might not support ignition. A typical example of this would be the case where the mixture was marginal for combustion (usually lean) and the velocity fluctuations large. If there is a high velocity fluctuation at the spark plug during the spark, the mixture will not ignite, whereas the velocity nearby might be lower and suitable for ignition using a large volume source.

The second possibility for ignition improvement is that of lower energy ignition. Considerable energy is wasted in creating and maintaining the arc column in a traditional spark plug, and it is possible that a low energy ignitor could be made to provide energy only into the chemical reaction channels needed to begin combustion. To demonstrate this a calculation of the minimum absorbed energy needed for combustion initiation would be done. A more efficient VUV generation source would also need to be found, since arc-generated radiation is obviously no improvement over an spark.

Magnetic Field Effects - Recent publications in the area of magnetic enhancement of combustion [32,33] indicate the status of this research. These works confirm many earlier statements in the literature that all of the obvious effects of electromagnetic fields on flames result from aerodynamic effects - that direct forces are exerted on the gases through the interaction with the plasma in the flame front. In the case of internal combustion engines, these aerodynamic effects must be used to increase the flame speed of the initial flame kernel. For maximum enhancement of flame speed the forces must primarily promote radial expansion from the spark plug. As suggested by Wakayama [33] a decreasing magnetic field away from the spark plug should lead to enhanced outward flow; a small, strong magnetic pole at the spark plug would have the most effect. A flame force could also be developed by inducing a current in the flame in an appropriately oriented magnetic field. One hazard of large magnetic fields in an internal combustion engine is the incidental induction heating of the moving piston. This heating should be estimated for any magnetic field experiment, since it may add sufficient heating to that derived from combustion to overload the cooling capabilities of the engine and lead to catastrophic failure - the piston may seize.

6. Energy Fields in Engine Experiments

The general conclusion of an analysis of what part of the operating envelope of an internal combustion engine is most amenable to improvement is that lean combustion and idle operation are most in need of improvement. For any engine test it is important to use a crank angle timing unit. Such a unit permits combustion effects to be initiated at a constant and repeatable time in the cycle for a duration that is a constant fraction of the cycle. Crank angle timing is important because under the influence of almost any change engine speed will change, changing the absolute time of both events and the time separating events, changing the effect on the cycle and the repeatability of the experimental conditions.

Research into the effects of energy fields in flames suggests some specific initial experiments in an engine. The point of these initial engine tests would be twofold: 1) Demonstrate that there is a working experimental setup that injects microwave energy into an operating engine cylinder, and 2) Demonstrate that a major beneficial effect on engine operation is caused by injecting the microwave energy. These tests are intended to provide a focus for initial experimental work, an experimental foundation on which to base further work, and an elegant demonstration for marketing to obtain continuing funding.

The tests center on the known effect of microwaves on ignition. Aside from the fact that microwaves alone can ignite a mixture, high power microwaves can contribute energy to the combustion that is comparable with the chemical energy during the initial stages of combustion. If this is the case the initial post-flame gases are hotter and expand faster as the flame burns. The added expansion makes the initial flame expand faster so that the turbulent flame area grows faster, leading to a significantly shorter period for initial combustion.

The importance of this is in relation to lean combustion, where the equivalence ratio is low enough for the overall combustion to be only marginally fast enough. The limiting effect of lean combustion is that some cycles cannot complete combustion, leading to unstable engine operation giving very reduced power output.

It is not clear how a specific engine will respond to lean combustion. For a side-valve engine, the combustion will be more sensitive to the slowing of combustion because the flame has much farther to travel than in a conventional central spark ignition engine. This will probably be the major effect (which we will use to advantage), although the flows around the spark plug may be such that lean engine operation also is sensitive to misfires.

A set of possible experiments, ranked in the order of their likelihood of success and the ease of performance is given below.

The engine is first set up to operate as lean as possible at idle until it starts running roughly. The best spark advance, fuel flow, and air/fuel ratio (equivalence ratio) should be measured if possible. The optimum microwave conditions are not obvious. The TM₀₁₀ resonant mode seems to be the best as a result of its insensitivity to the vertical dimension of the chamber. The piston is constantly changing this dimension, although in this engine it will be constant near Top-Dead-Center (TDC). Ward and Wu [34] perform a detailed analysis of the microwave response of in-cylinder combustion, which is probably mostly but not totally correct.

Power deposition must be controlled so as not to overpower the mixture and ignite the whole cavity at once (Detonation). For very lean mixtures this will probably not damage the engine, but DON'T try this with a stoichiometric mixture! The microwave power should be increased gradually instead of being applied at maximum power to determine if there is any effect. Any way to increase the power deposition locally would be a superior experimental technique. Volume ignition would work much better if 99% the microwave power was deposited in 10% of the volume.

Experiment #1 - Microwave combustion acceleration:

Add a separate microwave feedthru (or combine it with the spark plug, but allow the spark to ignite the mixture). Turn on the microwave generator when the spark fires (or leave it on steady state if the heating is not too bad) and leave it on for the entire initial part of combustion - at least from the spark through TDC. Start with low power levels while the engine operates at steady state, and steadily increase the power. At some power level the engine should start to run better. Record this power level. Then, leaving the microwaves on, give the engine less fuel until it starts running rough again, then increase the microwave power again. See how far this process can be iterated. You will have to decrease the spark advance when the microwaves begin to have an effect and increase the initial combustion speed. Without the microwaves, decreasing the spark advance will make the engine immediately run worse.

An excellent demonstration experiment for combustion enhancement by microwaves would be to have the engine running rough, then add the microwaves and make it operate smoothly. Next increase the microwave power, increase the spark advance and explain that the engine is now operating fine at conditions where it would normally not operate at all. At this point the microwaves would be shut off and the engine would immediately stall.

This demonstration is a powerful incentive to leave the spark plug in and show a very obvious improvement in normal engine operation using microwaves. Most experiments will have much less obvious effects with no clear cause and effect relationship.

Experiment #2 - Microwave ignition and combustion acceleration:

Same as above, but attempt to use the microwaves to ignite combustion also.

Experiment #3 - Microwave ignition to enhance initial flame size:

Use microwave energy to ignite the mixture over a much larger area than a conventional spark plug does. It is not clear how one would verify this effect short of combustion photography, but the procedures would be similar to that used for experiment #2. THis should be a smaller effect than in experiments #1 or #2, depending on how large the initial volume of ignition is. It cannot be the entire cylinder volume, of course; that would cause a pressure rise at the wrong place in the cycle. Once again, this engine configuration is helpful because the microwave energy will tend to be concentrated over the valves in one part of the cylinder volume.

Experiment #4 - Microwave large area ignition to prevent misfires:

Use microwave energy to ignite the mixture over a much larger area than a conventional spark plug does. Run the engine so lean that there are misfires. Turn up the microwave energy (short pulse) until the misfires disappear. Turn up the microwave energy more and see if misfires can be avoided using even leaner mixtures.

Both experiments 3 and 4 have problems associated with starting the engine. The difficulty is that a cold engine works very differently than a hot one, and steady state must by definition be reached with a hot engine. The microwave setup must somehow be adjusted to handle the change in engine conditions. Typically the engine will start with a choke of some kind, which means that the initial mixture is much more fuel rich versus when the engine has warmed up. You may be able to start the engine in a similar manner to that used with a spark plug or you may not. The behavior of ignition of a much richer cold mixture using microwaves would be expected to be very different from that of a hot lean mixture. At the very least, the breakdown conditions would be very different just in terms of gas density and temperature.

Other Experiments

VUV/UV Ignition - One simple experiment that might be done is to use an evacuated Xenon arc source near a sapphire window on the combustion chamber to generate significant Vacuum Ultraviolet (VUV) radiation inside the gas near the window as an ignition source. A higher VUV radiant flux can be achieved by focusing the radiation from a deuterium arc through a small MgFl₂ window with an elliptical reflector. Very efficient VUV reflectors can be made, and MgFl₂ has much better VUV transmission than sapphire. The low strength of MgFl₂ can be tolerated by using small windows. The disadvantage of the small window would be the small area ignition source.

One possible problem is that the absorption length of the radiation might be short enough for wall quenching to be an important negative effect. This radiation will ionize oxygen (but not nitrogen) close to the window, acting as an ignition source. There is a possibility that once all of the local oxygen is ionized the radiation can penetrate further, but this seems unlikely since although nitrogen does not photoionize, it does absorb VUV to become an excited molecule. Hydrocarbons will absorb and ionize also, but these molecules are in a much lower concentration than oxygen or nitrogen (for gasoline). All of the energy added to the air/fuel gas mixture should ignite the mixture.

Experiment Modifications for Combustion Enhancement Diagnosis

The first modification proposed is to use an Argon-Oxygen mixture instead of an air intake. Replacing the nitrogen in air with argon should not significantly affect normal engine operation, but it will remove nitrogen as the primary sink of microwave energy in the gas. Oxygen will then be the primary recipient of microwave heated electron excitation. If flame acceleration results from excited oxygen, this alternate gas composition will reveal the effect even if similar applied microwave power levels in air do not. The use of this oxidizer will probably also result in less ohmic heating and less absorbed power, perhaps leading to a determination of whether combustion acceleration obtained in these two cases results from ohmic heating or chemical kinetics enhancement.

The second modification is to add a window to the microwave channel to be able to detect optical emissions from the engine cylinder that result from microwave excitation. Since so much energy is being added to the gas there will almost certainly be an enhancement of radiant emission processes already taking place in the flame as well as the creation of different processes that will be detectable. The usefulness of optical diagnosis is that detection of specific radiation wavelengths will imply specific molecular excitation processes, and a knowledge of these excitation processes will permit insight into the microwave energy cascade and its kinetics in the gas. Such a knowledge might be crucial in adapting the microwave or other excitation process to achieve a useful modification of engine performance. One aspect of the radiation may also be important - it may be in the infrared, requiring both special detectors and windows (versus eyes and glass) to make its presence obvious.

Requirements for the Duration of Energy Field Excitation

It is important to determine the minimum duration of the application of the energy field that will result in a desired combustion enhancement. The required energy field pulse length might determine the total energy deposited in the process, and may determine whether a particular form of excitation is practical. The total deposited energy together with the needed frequency of application will determine the energy efficiency of the process relative to combustion heat release, and will partially determine the economics of the enhancement. The overall economic practicality of the process is determined by balancing the savings obtained from the overall engine redesign resulting from improved operation with the cost of the device that provides this improvement.

It must be emphasized that the pulse length that is required to achieve combustion enhancement depends both on the type of enhancement that is desired and on the mechanism of the enhancement.

The specific case discussed here is that of accelerating combustion at engine idle speed. This acceleration shortens the total combustion time and prevents the incomplete combustion that would otherwise occur. The benefit of this to be able to operate at a leaner operating condition than would otherwise be possible, because incomplete combustion of any type is not permissible as a result of pollution regulations. Leaner operation leads to a significant increase in fuel efficiency by permitting lower fuel consumption during idle. Idle vibration would also decrease, which is an important (but difficult to quantify) improvement in overall car quality from the consumer's viewpoint.

Accelerating combustion throughout a lean combustion cycle is most easily done by accelerating the initial phase of combustion. Incomplete combustion in a cycle (also known as a partial burn) is usually caused by a weak initial flame kernel [35-37], where the early flame kernel encloses a small volume as a result of internal flows that move it in such a way that its development is inhibited. There are three ways to increase the propagation rate of this early flame: 1) The local flame speed can be increased by increasing chemical kinetic rates in the flame, 2) The flame speed can be increased by ohmic heating in the flame front, or 3) the flame can be expanded by heating the gases that are already burned. Expanding the flame by heating the burned gases causes an increase in flame area and an increase in the compression of the remaining charge, both of which increase the subsequent combustion rate.

The simpler case to be discussed is that of burned gas heating. The electron temperature measurements of MacLatchy [9] indicate that additional microwave heating occurs in the burned gases behind the flame. This is consistent with the possibility that microwave flame enhancement is caused by ohmic heating, as claimed by Groff and Krage [15]. If ohmic heating is the primary cause of flame speed increase, the time of power deposition is unimportant; it is only important how much total energy is deposited and what total temperature increase results from the heating. If only the energy absorbed in the gas is important it is possible that a range of different energy transfer processes can be accessed by using different pulse lengths, and by selecting an optimum pulse length a more efficient energy deposition process might be achieved. In another context, a short pulse length may be preferred as a result of the type of equipment used - this type of equipment would be appropriate for ohmic heating but not reaction rate enhancement.

The second case to be considered is flame acceleration through artificially enhanced chemical reaction rates. Flame acceleration must take place before turbulence controls the global flame speed, which is very soon after ignition. Combustion enhancement also requires that the enhancement effect be maintained long enough to obtain a significant increase in flame area as the flame propagates. The object is to achieve a relative increase in flame area compared with the flame area that would occur at the same time in the cycle that occurs without enhancement. This implies that the duration of the enhancement will depend on the size of the flame kernel at the time of initial enhancement, the increase in flame speed, and the original flame speed. The largest relative increase in flame area occurs at the earliest time in the cycle, simply because the flame speed is constant and the flame area is increasing roughly with the square of the radius of the flame kernel. Acceleration of the flame for about 5 degrees crank angle (deg-CA) the engine is probably needed to accelerate combustion in the overall cycle. At 600 rpm each crank angle lasts for about 1 ms, implying a need for a pulse length of about 5 ms. An increase in flame speed of 100% caused by enhanced reaction rates is probably the best that one can expect, but simultaneous heating of burnt gases behind the flame would reduce the needed enhancement time if both effects are of comparable magnitude. Burnt gas expansion coupled with intensification of the flame might be able to advance the flame propagation by 5 deg-CA in perhaps only a fraction of a deg-CA. In any case the energy applied must be minimized to make the overall process energetically feasible.

The final case of flame speed enhancement through local ohmic heating in the flame front will simple add to the chemical enhancement effect and obey the same duration requirements because it is a local effect.

7. Energy Fields in Engines - Questions

The following are some of the questions that have arisen in the course of research and should be addressed in the course of future research. The questions are not listed in order of importance.

What is the response of the engine cylinder with combustion and piston movement to microwave excitation?

How much of the incident microwave power is dissipated in the cavity (cylinder) walls? What is the skin depth?

What is the dependence of electron density on fuel characteristics?

What fuel additives can be used to increase electron density in the flame?

Are there other applications for energy fields in combustion and pollution abatement.

How much of the incident microwave power is dissipated in the cavity (cylinder) walls? What is the skin depth?

Applying energy fields to lean flames should lead to bigger changes than for stoichiometric flames, but ne decreases also, is there some optimum?

What is the relaxation time of an artificially elevated electron temperature?

Do diesel flames have similar electron densities to SI engines?

There are a number charged particle effects in soot production, can these be changed by energy fields for some benefit in sooting combustion?

Is it necessary to use the energy field intermittently or can they be used in every cycle?

Are there optically observable results of the energy deposition so that the energy transfer processes can be verified or identified?

8. Energy Fields in Engines References

1. R.M. Fristrom and A.A. Westenberg, Flame Structure, McGraw-Hill, New York, (1965)

2. C.K. Westbrook, and F.L. Dryer, "Chemical Kinetic Modeling of Hydrocarbon Combustion," Prog. Energy Comb. Sci., 10, 1-57, (1984).

3. F.A. Smith, "Problems of Stationary Flames," Chem. Rev., 21, 389 (1937).

4. R.M. Fristrom, C. Grunfelder, and S. Favin, "Methane-Oxygen Flame Structure. I. Characteristic Profiles in a Low Pressure Laminar Lean Premixed Methane-oxygen Flame," J. Phys. Chem., 64, 1386, (1960).

5. A.A. Westenberg, and R.M. Fristrom, "H and O Atom Profiles Measured by ESR in C₂ Hydrocarbon-O₂ Flames," Tenth Symp. (Intl.) on Combustion, The Combustion Institute, Pittsburgh, (1965).

6. H.C. Jaggers, and A. von Engel, "The Effect of Electric Fields on the Burning Velocity of Various Flames," Comb. and Flame, 16, 275-285, (1971).

7. H.F. Calcote, Eighth Symp. (Intl.) on Comb., The Combustion Institute, Pittsburg, 181-199, (1962).

8. Advanced Combustion Methods, F. Weinberg, Ed., Academic Press, New York, (1986).

9. C.S. MacLatchy, R.M. Clements, and P.R. Smy, "An Experimental Investigation of the Effect of Microwave Radiation on a Propane-Air Flame," Combust. Flame, 45, 161-169, (1982).

10. D. Bradley, and S.M.A. Ibrahim, Electron-Gas Energy Exchanges in ac Fields and Their Relevance in Lasers," Comb. and Flame, 27, 353, (1976).

11. D. Bradley, and S.M.A. Ibrahim, "The Effects of Electrical Fields upon Electron Energy Exchanges in Flame Gases," Comb. and Flame, 22, 43, (1974).

12. R.A. Strehlow, Combustion Fundamentals, McGraw-Hill, New York, (1984).

13. G. Wortberg, Tenth Symp. (Intl.) on Comb., The Combustion Institute, Pittsburgh, 651-655, (1965).

14. A.C. McIntosch, and J.F. Clarke, Combustion in Reactive Systems, 76, Prog. in Astro. and Aero., AIAA, 443-462, (1981).

15. E.G. Groff, and M.K. Krage, "Microwave Effects on Premixed Flames," Comb. and Flame, 56, 293-306, (1984).

16. H.C. Jaggers, "Electric Studies of Combustion Processes," PhD Thesis, Univ. of Oxford, (1969).

17. J.O. Hirschfelder, C.F. Curtiss, and R.B. Bird, Molecular Theory of Gases and Liquids, Chapman and Hall, London, 21, (1954).

18. A. Von Engel, Brit. J. Appl. Phys., 18, 1661, (1967).

19. L.P. Hurle, J. Chem. Phys., 41, 3592, (1964).

20. L.P. Hurle, "The Coupling between Free Electron and Molecular Vibrational Temperatures in Plasma Environments," Symp. on the Prop. of Low-Temp. Plasmas, Moscow, (1965).

21. G.J. Schulz, Phys. Rev., 116, 1141 (1959): ibid., 125, 229 (1962): ibid., 135, A988 (1964).

22. K. Kleinermanns, and R. Schinke, "Dynamics of H + O₂ → OH + O at high collision energies," J. Chem. Phys., 80, 4, 1440-1445, (1984).

23. Lewis and Trainer, AVCO Everett Report #AMP 422, under ARPA Contract # F04701-73-C-0284, Nov. (1974).

24. O.C. Ellis, and W.A. Kirkby, Flame, Methuen, London, 47, (1936).

25. J.C. Palanyi, and W.H. Wong, "Location of Energy Barriers. I. Effect on the Dynamics of Reactions A + BC," J. Chem. Phys., 51, 1439, (1969).

26. J.C. Palanyi, and M.H. Mok, "Location of Energy Barriers. II. Correlation with Barrier Height," J. Chem. Phys., 51, 1451, (1969).

27. R.M. Fristrom, "Survey of Progress in Chemistry," Academic, New York, 89, (1966).

28. A. von Engel, Ionized Gases, 2nd Ed., Oxford Univ. Press, London, 126, (1965).

29. A. von Engel, and J.R. Cozens, International Symp. on Fundamental Studies of Ions and Plasmas, 1, 123, AGARD: Pisa (1965).

30. R.M. Clements, R.D. Smith, and P.R. Smy., "Enhancement of Flame Speed by Intense Microwave Radiation," Comb. Sci. and Tech., 26, 77-81, (1981).

31. W.J. Miller, Fourteenth Symp. (Intl.) on Comb., The Combustion Institute, Pittsburg, 307-320, (1973).

32. T. Pedersen, and R.C. Brown, "Simulation of Electric Field Effects in Premixed Methane Flames," Comb. and Flame, 94, 433-448, (1993).

33. N.I. Wakayama, "Magnetic Promotion of Combustion in Diffusion Flames," Comb. and Flame, 93, 207-214, (1993).

34. M.A.V. Ward, and T.T. Wu, "A Theoretical Study of the Microwave Heating of a Cylindrical Shell, Flame-Front electron Plasma in an Internal Combustion Engine," Comb. and Flame, 32, 57-71, (1978).

35. S.C. Bates, "Insights into Spark-Ignition Four-Stroke Combustion Using Direct Flame Imaging," Comb. and Flame, 85, 3 & 4, 331-352 (1991).

36. S.C. Bates, "Flame Imaging Studies of Flame Development in a SI Four Stroke Engine," Dynamics of Deflagrations and Reactive Systems: Flames, A.L. Kuhl, J.C. Leyer, A.A. Borisov, and W.A. Sirignano, Progress in Astronautics and Aeronautics, 131, AIAA, Washington, DC, 335-377 (1991).

37. S.C. Bates, "Flame Imaging Studies of Cycle by Cycle Combustion Variation in a SI Four Stroke Engine," SAE paper 892086 (1989).

Home